Binary compact objects such as black holes and neutron stars merging billions of years ago emit gravitational waves that can be detected with the detectors operated by the LIGO–Virgo–KAGRA Collaborations. Einstein taught us that gravity curves space and time, affecting everything that carries mass or energy, and gravitational waves are no exception. Therefore, we expect that as they travel cosmological distances, gravitational waves will inevitably encounter massive astrophysical objects that would act as lenses, bending their trajectories and even distorting the shapes of their signals.
In this study, we have searched for the still undetected lensing signatures in the gravitational-wave signals detected in our latest LIGO-Virgo-KAGRA catalog, which includes events from the full third observing run (O3). This work extends our previous analysis of the first half of O3.
What Are The Signatures Of Gravitational Lensing Of Gravitational Waves That We Are Searching For?
Gravitational lenses are very different from usual lenses, and the phenomenology of gravitational lensing of gravitational waves is broad and rich. When a gravitational wave signal travels close enough to a galaxy or galaxy cluster it may be strongly lensed, producing multiple images of the original signal, see Fig. 1. In this regime of strong lensing each image travels along a different trajectory, feeling a different gravitational potential, and thus arriving at the detector at different times and with a different magnification. Since the shape of the signal is mostly unchanged by strong lensing, we search for these repeated events in our catalog by looking for signals that coincide in their detected masses and other intrinsic parameters. We perform this search in different stages, first using rapid algorithms that look for similarities in the inferred parameters for different events in order to cull down the list of candidates. We then perform sophisticated statistical analyses to determine the significance of the strong lensing hypothesis versus the non-lensing hypothesis. Since some of the images could be de-magnified falling below our detection thresholds, we also perform targeted searches of sub-threshold images. Note that when a gravitational wave signal travels not so close to the galactic lens, the effect is only a weak magnification or de-magnification of the emitted signal. Therefore, we are currently not searching for this weak lensing regime, although it will be important in the future when more distant binaries are detected.


Figure 1: Schematic representation of the gravitational lensing of gravitational waves. On the top, a given signal is strongly lensed by a galaxy lens producing multiple images with different amplitude, arrival time and phase. On the bottom, small compact lenses produce interference effects that distort the detected waveform.
But galaxies and clusters of galaxies are not the only objects that could lens gravitational waves. Much smaller astrophysical objects such as isolated black holes could also lead to detectable lensing effects. Since the time delay between the multiple images is proportional to the mass of the lens, lighter lenses would lead to shorter time delays. Eventually, when the time delay is less than the duration of the signal, the images may overlap producing interference effects visible as beating patterns in the waveforms, see Fig. 1. For even smaller masses, diffraction effects may be important and lensing must be solved in the full wave-optics regime. However, if the lens is much smaller than the gravitational wavelength, no significant effect can be measured. In any case, this means that LIGO–Virgo–KAGRA sources can probe lenses of hundreds to tens of thousands of solar masses, as those may imprint detectable waveform distortions. Following our previous study, we search for these “beating patterns” in the data.
What New Analyses Have We Implemented?
In this study we have updated and improved our previous work, but we have also introduced new analyses. In particular, a new rapid pipeline to identify strongly lensed candidate pairs has been introduced. Instead of looking at overlap in parameters, this new pipeline uses machine learning to compare directly the similarity in the morphology of the time-frequency spectrograms. Since strong lensing mostly leaves the shape of the images unchanged, spectrograms should remain similar for all images. In this study we have used this machine learning technique in conjunction with the posterior overlaps to select the most interesting candidates. For those we perform a more computational expensive joint parameter estimation that leads to no evidence of strong lensing (see Fig. 2).
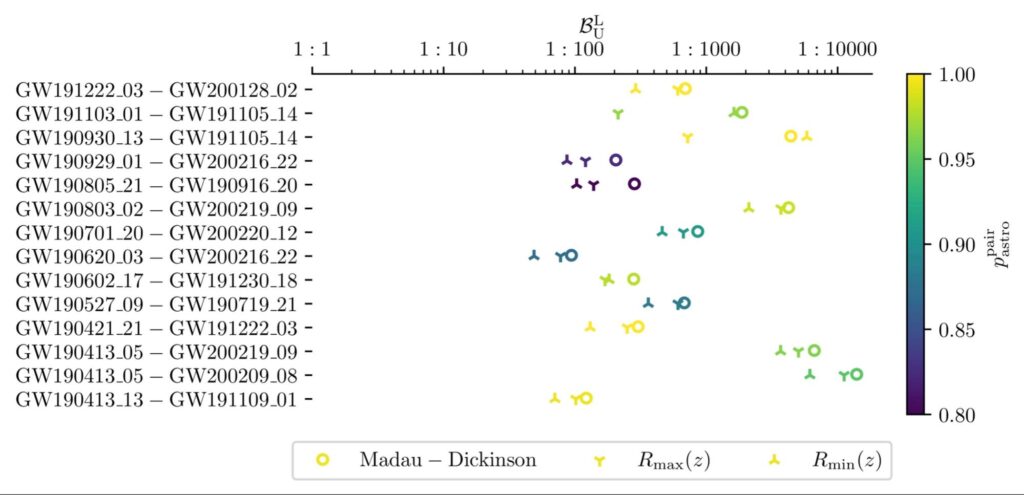
Figure 2: Summary plot for our search of strongly lensed gravitational waves. For each pair of candidate lensed events listed in the left column, we compute the statistical evidence of the lensed versus non-lensed hypothesis (BUL). A BUL larger than 1 indicates preference for the lensed hypothesis. The horizontal axis indicates this evidence starting at 1, with decreasing probability to the right. We compute this evidence for different assumptions about the merger rate history of binary black holes, summarized by the three different symbols that encompass a merger rate following the star formation rate (“Madau-Dickinson”), and the minimum and maximum of a compilation of astrophysical simulations. For reference we also add a color bar that indicates the probability that this pair has an astrophysical origin independent of the lensing scenario. None of the pairs show preference for the lensing hypothesis.
As mentioned before, strong lensing mostly leaves all image shapes unchanged. For some of them, known as type II images, there could be some small distortions when different frequency modes are relevant. In this study we search for these signatures of strong lensing in individual events. Although some candidates show mild evidence of this subtle effect, a detailed follow-up analysis does not provide conclusive evidence of strong lensing.
Implications From Not Finding Any Gravitational Wave Lensing
The fact that we do not find evidence of lensing allows us to draw astrophysical implications on the population of lenses and sources. Multiple-image searches constrain the distribution of large lenses such as galaxies, while interference effects set limits on the amount of compact objects acting as the dark matter. For the latter, assuming that all compact dark matter objects have the same mass, we can set limits on their abundance for different masses, see Fig. 3. This in turn has implications for early Universe models predicting the existence of primordial black holes. As it can be seen in the figure, we find that compact objects with masses between 100 and 10,000 solar masses could not be the totality of dark matter. For the former, we find that the non-observation of strong lensing implies that the merger rate of binary black holes in the distant past (more than 10 billion years ago) could not be more than two orders of magnitude larger than the merger rate at present time, or otherwise we should have observed multiply lensed events. This result is consistent with upper bounds derived by the non-observation of a stochastic gravitational wave background.

Figure 3: Constraints on the abundance of compact objects derived from the non-observation of lensing distortions in the latest gravitational wave catalog. The abundance of compact objects on the vertical axis is computed as a fraction of the total dark matter abundance. The mass of the compact objects on the horizontal axis is presented in terms of the mass of the lens. We consider different assumptions, partly summarized by the ‘flat prior’ and by the ‘Jeffreys prior’, for the population of lenses and sources. We find that compact objects between 100 and 10,000 solar masses could not be the totality of the dark matter.
The Road Ahead
Although we have not found compelling evidence of lensing, the improved sensitivity in the upcoming observing runs places gravitational wave lensing searches in a very exciting position at the edge of its first detection.
Find Out More:
- Visit our websites:
- Read a free preprint of the full scientific article here or on arXiv.org.
Back to the overview of science summaries.

